I first encountered symbolic
logic when I was a teenager. Unfortunately, I had great trouble following the ostensible explanations that I encountered, and I didn't recognize that my perplexity was not because the underlying subject were intrinsically difficult for me, but because the explanations that I'd found simply weren't very well written. Symbolic logic remained mysterious, and hence became intimidating. And it wasn't clear what would be its peculiar virtue over logic expressed in natural language, with which I was quite able, so I didn't focus on it. I was perhaps 16 years old before I picked-up any real understanding of any of it, and it wasn't until years after that before I became comfortable not simply with Boolean expression but with processing it as an algebra.
But, by the time that I was pursuing a master's degree, it was often how I generated my work in economics or in mathematics, and at the core of how I presented the vast majority of that work, unless I were directed otherwise. My notion of an ideal paper was and remains one with relatively little natural language.
Partly I have that notion because I like the idea that people who know mathematics shouldn't have to learn or apply much more than minimal English to read a technical paper. I have plenty of praise for English, but there are an awful lot of clever people who don't much know it.
Partly I have that notion because it is easier to demonstrate logical rigor by using symbolic logic. I want to emphasize that word demonstrate
because it is possible to be just as logically rigorous while expressing oneself in natural language. Natural language is just a notation; thinking that it is intrinsically less rigorous than one of the symbolic
notations is like thinking that Łukasiewicz Polish notation is less rigorous than infixing notation or vice versa. I'll admit that some people may be less inclined to various sorts of errors using one notation as opposed to another, but which notation will vary amongst these people. However, other people don't necessarily see that rigor when natural language is used, and those who are inclined to be obstinate are more likely to exploit the lack of simplicity in natural language.
But, while it may be more practicable to lay doubts to rest when an argument is presented in symbolic
form, that doesn't mean that it will be easy for readers to follow whatever argument is being presented. Conventional academic economists use a considerable amount of fairly high-level mathematics, but they tend to use natural language for the purely logical work.[1] And it seems that most of them are distinctly uncomfortable with extensive use of symbolic logic. It's fairly rare to find it heavily used in a paper. I've had baffled professors ask me to explain elementary logical transformations. And, at least once, a fellow graduate student didn't come to me for help, for fear that I'd immediately start writing symbolic logic on the chalk-board. (And perhaps I would have done so, if not asked otherwise.)
The stuff truly isn't that hard, at least when it comes to the sort of application that I make of it. There is a tool-kit of a relatively few simple rules, some of them beautiful, which are used for the lion's share of the work. And, mostly, I want to use this entry to high-light some of those tools, and some heuristics for their use.
First, though, I want to mention a rule that I don't use. 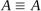 This proposition, normally expressed in natural language as
This proposition, normally expressed in natural language as A is A
and called the Law of Identity
, is declared by various philosophers to be one of the three Principles of logic. But I have no g_dd_mn'd idea what to do with it. It's not that I would ever want to violate it; it's just that I literally don't see anything useful to it. Ayn Rand and many of those for whom she is preceptrix treat it as an essential insight, but I think that it's just a dummy proposition, telling me that any thing can stand where that thing can stand.[2]
[1] There's an idiotic notion amongst a great many mainstream economists that the Austrian School tradition is somehow less rigorous simply because some of its most significant members eschew overt mathematics in favor of logical deduction expressed in natural language. But most of the mainstream is likewise not using symbolic
logic; neither is necessarily being less rigorous than otherwise. The meaning of variables with names such as qt
can be every bit as muddled as those called something such as the quantity exchanged at this time
. There are good reasons to object to the rather wholesale rejection of overt mathematics by many Austrian School economists, but rigor is not amongst the good reasons.
[2] [Read more.]