Next!
13 April 201114 A corroboration of this point of view seems to come from some of the so-called tests for logical thinking, which include questions such as
What is the next number in the (!) sequence 1, 3, 6, 10 … ?and (in slightly simplified form)Which of the following four figures differs from the other three: a square; a cross; a circle; a triangle?Questions of this kind do indeed test a valuable ability, viz. the ability to guess what the man who formulated them had in mind, e.g. the circle, because it is the only figure that is round (although, of course, the square is the only one with four corners; the cross the only one with a ramification point; the triangle the only one with exactly three corners). What in this way certainly is not tested is logical thinking. Yet the marks youngsters make in such tests highly correlate with their achievements in elementary mathematics! Far from demonstrating that those test questions have more to do with logic than with guessing and empathy this correlation rather seems to indicate that the presentation of elementary mathematics has more to do with guessing and empathy than with logical thinking.
foot-note
Austrian Marginalism and Mathematical Economics (1971)
in Carl Menger and the Austrian School of Economics (1973)
edited by John Richard Hicks and Wilhelm Weber
(Karl Menger, an eminent mathematician of the 20th Century, was the son of Carl Menger, one of the preceptors of the Marginal Revolution in economics, and founder of the Austrian School of economics.)

Interesting...I would have said the cross because it is the only one that isn't an enclosed form, like the other three. It goes to show people think along differing points of reference. But I see your point about the circle, therefore in my mind both answers would be correct in some completely defensible and logical fashion. It goes to show how easily human beings can misunderstand each other when we express facts as interpreted from varied points of view.
please excuse my typos...
I've fixed those that I spotted as such.
I previously installed a plug-in that is supposed to give the writers of comments an opportunity (for a limited time) to fix their comments. But one had to be logged into the website for it to work, and I don't know whether it now works at all. (There have been multiple changes to other things since last I tested it, and those changes might have broken the plug-in.)
The point here (which is by way of Karl Menger) is that the only real error is on the part of the questioner.
Given any set S, and any item x as a candidate for inclusion in a superset of S, we can always find a rule Ri that fits the elements of S and includes x, and always some other rule Re that fits the elements of S but excludes x.
I mean that without exception. Sometimes one would have to do a lot of work to find Ri or Re, but they always exist. In some cases, a mediocre mind does not find them, and concludes that they must not exist, while a clever mind sees them. If the mediocre mind is that of an educator, and the clever mind is that of a student, then not only will there be the communication problem that you note, but the student is likely to be treated as a dullard who cannot see the obvious.
The problem is perhaps most acute when the clever mind has not yet been shown (or recognized) the general principle here. (So, for example, bright children are led to believe that they are failing to see something logical, when in fact they are just seeing more than do those around them.) But I once had an argument about the specific case of a particular sequence of integers, with someone who had a doctorate in engineering from UCSD, and thus should have seen the math. My knowing and articulating the principle wasn't sufficient for me to get him to see his error. (He demanded that I find the quasi-algebraic generating function for a specific case; I told him that there would be a fee for that service; his response was insult.)
15, but only because nothing else works within a few minutes of logical thought. And the cross, it's the only design where one has to lift their pencil to complete (without re-tracing a line) 😉 I've never considered such questions as a true measure of logic. x = 2 + 2 is logic.
The first alternative sequence that occurs to me has the differences of the differences generated as the Fibonacci Sequence.
Your point on the cross is mathematically very close to that made by Menger. As a tangent: I was surprised that his thought in using the word was — and the interpretation of at least two of my readers has been — simply two crossing line segments. In the context of the other figures, my thoughts went first to a polygon such as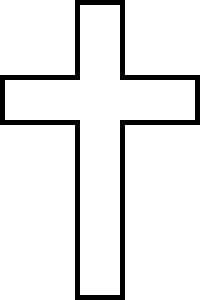 (which plainly has no ramification point and can be drawn without lifting a pencil or retracing a line). I would note that this figure, unlike the square, circle, and triangle, is not convex, which is to say that it is possible to connect some pair of points on its perimeter with a line segment that it does not contain.
(which plainly has no ramification point and can be drawn without lifting a pencil or retracing a line). I would note that this figure, unlike the square, circle, and triangle, is not convex, which is to say that it is possible to connect some pair of points on its perimeter with a line segment that it does not contain.