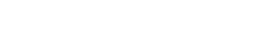l'usage
Thursday, 3 January 2019In the course of a present investigation of how the main-stream of economics lost sight of the general concept of utility, I looked again at the celebrated article Specimen Theoriæ Novæ de Mensura Sortis
by Daniel Bernoulli, in which he proposed to resolve the Saint Petersburg Paradox[1] by revaluing the pay-off in terms of something other than the quantity of money.
The standard translation of his article into English[2] replaces Latin emolument-
everywhere with utility
, but emolumentum
actually meant benefit.[3] Bernoulli's own words in his original paper show no more than that he thought that the actual marginal benefit of money were for some reason diminishing as the quantity of money were increased. However. before Bernoulli arrived at his resolution, Gabriel Cramer arrived at a resolution that had similar characteristics; and, when Bernoulli later learned of this resolution, he quoted Cramer. Cramer declared that money was properly valued à proportion de l' uſage
[in proportion to the usage
]. The term uſage
itself carries exactly the original sense of utility
. (Cramer goes on to associate the usefulness of money with plaiſir
, but does not make it clear whether he has a purely hedonic notion of usefulness.) Bernoulli did not distinguish his position from that of Cramer on this point, so it is perfectly reasonable to read Bernoulli as having regarded the actual gain from money as measured by its usefulness.
Of course, both Cramer and Bernoulli were presuming that usefulness were a measure, rather than a preördering of some other sort.
[1] The classic version of the Saint Petersburg Paradox imagines a gamble. A coin whose probability of heads is that of tails is to be flipped until it comes-up tails; thus, the chance of the gamble ending on the n-th toss is 1/2n. Initially, the payoff is 2 ducats, but this is doubled after each time that the coin comes-up heads; if the coin first comes up tails on the n-th flip, then the pay-off of the gamble will be 2n ducats. So the expected pay-off of the gamble is ∑[(1/2n)·(2n ducats)] = 1 ducat + 1 ducat + … = ∞ ducats Yet one never sees people buying such contracts for very much; and most people, asked to imagine how much they would pay, say that they wouldn't offer very much.
Cramer's resolution did not account for the preëxisting wealth of an individual offered a gamble, and he suggested that the measure of usefulness of money might be measured as a square root of the quantity of money. Bernoulli's resolution did account for preëxisting wealth, and suggested that the actual benefit of money were measurable as a natural logarithm.
I'm amongst those who note that one cannot buy that which is not sold, and who believe that people asked to imagine what they would pay for such a contract instead imagine what they would pay for what were represented as such a contract, which could not possibly deliver astonishingly large amounts of purchasing power.
[3] In a footnote, translator Louise Sommer claims that mean utility
is a free translation of emolumentum medium
and then that the literal translation would be mean utility
; I believe that she had meant to offer something else as the literal translation, but lost her train of thought.